
近几十年来,密度泛函理论(DFT)一直是第一性原理材料科学的主力军。改进的交换关联(xc)泛函如今已有数百种不同类型,包括广义梯度近似(GGA)、元GGA、(筛选的)混合函数、Hubbard修正的局部密度近似(LDA U)/广义梯度近似(GGA U)和非局域范德华(vdW)密度泛函等。通常,这些泛函包含针对特定类型或特定材料类别对几个参数所作的优化。此外,它们依赖于交换近似和关联近似之间的误差消除,限制了常用交换关联泛函的通用性和预测能力,其准确性也常常高度依赖于系统。这种非通用性的特点不能令人满意。
来自丹麦技术大学物理系计算原子尺度材料设计(CAMD)的Thomas Olsen教授带领的团队,重点综述了含时密度泛函理论(TDDFT)中的静态非局部交换关联泛函所描述的RPA和GW方法中的物理理论、实现途径和意义。作者并未详述RPA和GW方法本身,该方法的综述已在另一篇综述中阐明。在“理论”一节中,他们分别介绍了基于绝热连接涨落耗散定理和Hedin方程的基态和QP能量计算的基本理论。作者为HEG引入了几个非局域的交换关联泛函,并描述了从(半)局域交换关联泛函构造非局域交换关联泛函的重整化过程。
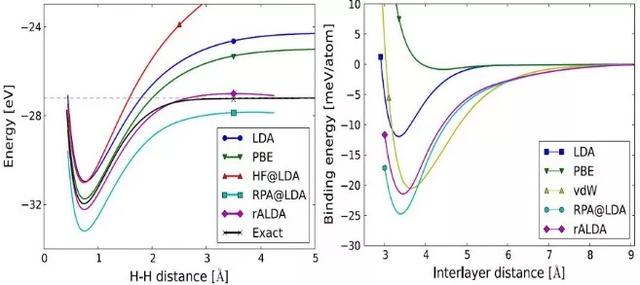
在“实现”一节中,作者描述了非局域交换关联泛函的数值实现,包括将HEG泛函推广到非均匀密度体系的不同策略、倒空间格点和基集收敛性等方面。在“结果”一节中,作者提供了一系列计算结果,用以说明交换关联泛函对总能量和QP能带结构的影响和重要性。具体而言,作者评估了重整化绝热局域密度近似(rALDA)和重整化绝热广义梯度近似(rAPBE)在固体结构参数、共价固体和共价分子的原子化能、氧化物形成能、vdW键、静态关联的原子二聚体的解离、表面和化学吸附能、结构相变,以及块体和二维半导体的QP能等方面的性能。最后,对全文进行了总结和展望。
该文近期发表于npj Computational Materials 5: 106 (2019),英文标题与摘要如下,点击https://www.nature.com/articles/s41524-019-0242-8可以自由获取论文PDF。
Beyond the RPA and GW methods with adiabatic xc-kernels for accurate ground state and quasiparticle energies
Thomas Olsen, Christopher E. Patrick, Jefferson E. Bates, Adrienn Ruzsinszky & Kristian S. Thygesen
We review the theory and application of adiabatic exchange–correlation (xc)-kernels for ab initio calculations of ground state energies and quasiparticle excitations within the frameworks of the adiabatic connection fluctuation dissipation theorem and Hedin’s equations, respectively. Various different xc-kernels, which are all rooted in the homogeneous electron gas, are introduced but hereafter we focus on the specific class of renormalized adiabatic kernels, in particular the rALDA (renormalized adiabatic local density approximation) and rAPBE (renormalized adiabatic Perdew–Burke–Ernzerhof). The kernels drastically improve the description of short-range correlations as compared to the random phase approximation (RPA), resulting in significantly better correlation energies. This effect greatly reduces the reliance on error cancellations, which is essential in RPA, and systematically improves covalent bond energies while preserving the good performance of the RPA for dispersive interactions. For quasiparticle energies, the xc-kernels account for vertex corrections that are missing in the GW self-energy. In this context, we show that the short-range correlations mainly correct the absolute band positions while the band gap is less affected in agreement with the known good performance of GW for the latter. The renormalized xc-kernels offer a rigorous extension of the RPA and GW methods with clear improvements in terms of accuracy at little extra computational cost.






